|

|
Nicolas Juillet
IRIMAS - Département de Mathématiques
18 rue des Frères Lumière
68 093 MULHOUSE
Tel : (00 33) 3 89 33 64 26
|

|
Announcement: proposal for a postdoc and a doctoral project starting from September 2025 inside the SOCOT project, Post-doc description, Doctoral thesis, Pre-doc/Master thesis. Here you'll soon also find the description for a post-doctoral researcher position (start 09/25 or 10/25).
Bienvenue sur ma page web! / Welcome
to my web page !
I'm a "Professeur des Universités" at Université de Haute-Alsace (UHA) in Mulhouse at IRIMAS, which is an institute made of three departments: computer science, mathematics, engineering. The mathematics department of IRIMAS is also part of the MaGE Federation together with the other research unit in the French region "Grand Est". On the period 2009--2021 I've been a "Maître de conférence" at "Université de Strasbourg" at IRMA. I'm the coordinator of SOCOT. My short CV 09/24 (French).
Research
- In this paper with Gaoyue Guo and Wenpin Tang we study the "soccer model" of Aldous and Kolesnik for tournaments and ranking in relation with the optimal transport techniques and its martingale version. .pdf
- I’m the last author of a paper in signal processing after a collaboration in interdisciplinary with Ali Moukadem, Barbara Pascal and Jean-Baptiste Courbot. Ali and Jean-Baptiste are members of the Automatics-Signal-Image (ASI) department of our common Institute (IRIMAS has three departments: maths, ASI and informatic). Barbara is a researcher at Centrale Nantes. ArXiv
- Joint publication with Augustin Fruchard (Mulhouse) and Rheinhard Schäfke (Strasbourg). The worst case for the (planar quadratic Euclidean) Traveling Salesman Problem is studied. Part of the paper is for clarification of this apparently difficult problem and for conjectures. But we have also results! Published in Bull. Math Soc. Sci. Math. Roumanie, 2024, No. 2. (special number in the occasion of Tudor Zamfirescu's 80th birthday) .pdf
- Joint publication with Moreno Andreatta and Corentin Guichaoua in complement to our paper on the generalized Hexachordal Theorem. Published in SIGMA 20, 009 (special number in the occasion of Jean-Pierre Bourguignon's 75th birthday) .pdf .DOI
- May 2021: In my second paper with Charles Boubel we specify what is the Markov-quantile process (that we introduced in the first paper) in the case of absolutely continuous curves in the Wasserstein space. Doing this we obtain a canonical process that is a minimizer solution of the Lagrangian continuity equation and is Markovian. Accepted for publication in Annales de l'Institut Henri Poincaré. Probabilités et Statistiques. .pdf.
- With Martin Brückerhoff we prove that the value function and the set of solutions of the martingale transport problems are not continuous from dimension 2. .pdf, DOI. Published in Electronic Communications in Probability, 2022, Vol. 27, paper no. 24, 1-10.
- Oct. 2020: In a side project with Moreno Andreatta and Corentin Guichaoua we revisit with metric measure spaces and probabilities the famous "Hexachordal Theorem", a surprising identity coming from the theory of music. To appear in Rendiconti del Seminario Matematico
della Università di Padova .pdf
- Collaboration with Martin Brückerhoff and Martin Huesmann. A peacock is a family of real measures indexed in a way the measures are increasing in convex order. Kellerer's theorem tells us that this is exactly a condition, so that there exists a martingale with these measures as marginals. Using ideas from martingale and stochastic optimal transport we show how to define in systematic way such a martingale. No special assumption is made on the initial measures. .pdf , DOI.Published in Electronic Journal of Probability Vol 27 (2022), paper no. 127, 62p.
- In this short paper written with Jean Bérard, we compare in convex order two sums of positive random variables with randomly many terms M and N. It is known that if M and N are in convex order, so are the corresponding sums. Our (new) proof is effective since it is based on a coupling. We also provide an algorithm .pdf. Published in Electronic Communications in Probability 2020, Vol. 25, paper no. 45, 1-9.
- The Monge problem on the real line can still be the subject of a paper! We select a uniquely determined solution approximating the problem by the problem for concave power costs .pdf. To appear in SIAM Journal on Mathematical Analysis.
- This is a proof that Brunn-Minkowski inequalities (and CD(K,N) conditions are not satisfies on subRiemannian structures) generalizing my thesis result to a more general geometric setting .pdf. To appear in Revista Matematica Ibroamericana.
- In this paper written with Nathael Gozlan we investigate a generalized transport problem .pdf. Published in Proceedings of the London Mathematical Society 120 (2020), no. 3, 434-463.
- In the following paper (joint work with Charles Boubel) we define the Markov-quantile process attached to a curve of probability measures. This has connections with the peacock problem and the transport equation from an optimal transport perspective .pdf. To appear in Journal de l'Ecole Polytechnique 9 (2022).
- In this short paper written with Jean Bérard, we examine the reconciliation of probability measures defined each on a sigma-algebra of the sape space Omega .pdf. Published in European Journal of Applied Mathematics 30 (2019), no. 6, 1300-1310 (special issue ``Applied Optimal Transport'').
- As new martingale optimal transports we introduce the shadow couplings, generalizing the left-curtain coupling. A connexion is made with the Skorokhod embedding problem. This is a joint work with Mathias Beiglböck, .pdf. To appear in Trans. Amer. Math. Soc.
- In this joint work with Mario Sigalotti we look at generalizations of the Whitney extension theorem for Carnot groups. We introduce the notion of pliable Carnot group .pdf. Published in Analysis & PDE, No. 7, 1637-1661 (2017).
- This following paper with Matthias Erbar is in relation with an optimal transport approach of the Ricci flow that makes sense for metric spaces. We look at Euclidean cones and the Heisenberg group .pdf. Published in Journal de Mathématiques Pures et Appliquées (9) 110 (2018), 123--154.
- A paper on examples/counterexamples for peacocks and martingales indexed on the plane .pdf. Published in Séminaire de Probabilités XLVIII, 13--32.
- Here is my paper about hypoelliptic diffusion seen from the
point of view of optimal transport, still for Heisenberg
groups. Published in Calculus of Variations and PDEs, 2014, Volume 50, Issue 3-4, pp 693-721. It is quite different from the original preprint of 2009 (.pdf)
- My small article on the structure of the Euclidean Wasserstein
space, entitled "On displacement interpolation of measures
involved in Brenier's Theorem" has appeared in Proc.
of AMS, 139(10) (2011). (.pdf)
- "A counterexample to the geometric traveling salesman problem
in the Heisenberg group" has appeared in the
Revista Matematica Ibroamericana, 26(3) (2010). It
presents a rectifiable curve that does not satisfy a criterion
that was supposed to be true for all rectifiable curves of the
Heisenberg group. (see .pdf)
- In a paper with Alessio
Figalli we answer an open question of L. Ambrosio and S.
Rigot (JFA 2004) about the optimal transport in the Heisenberg
group : during an optimal transport starting from an absolutely
continuous measure, the interpolated intermediate measures are
absolutely continuous too. This paper is published in Journal
of Functional Analysis, 255 (2008), no 1. see (.pdf)
- There are certain new synthetic Ricci curvature bounds whose
definition is adapted to the metric measure spaces. If you want
to know what happens when you consider them for the Heisenberg
group, you can have a look at Int.
Math. Res. Not. IMRN 2009, no. 13, 2347-2373. or (.pdf). NB: this paper is called
"Geometric inequalities and generalized Ricci bounds in the
Heisenberg group". It is sometimes still referred under its
preliminary name: "Ricci curvature bounds and geometric inequalities
in the Heisenberg group".
In December 2018, I've been defending my habilitation thesis "Transport optimal en théorie des processus et en géométrie" (other link).
In December 2008, I've been defending my doctoral thesis in
mathematics at the Institut Fourier (Université Grenoble 1) and
the Institut
für angewandte Mathematik (Universität Bonn). My advisors
were Hervé
Pajot and Karl-Theodor Sturm. My thesis is called "Optimal
transport and geometric analysis in Heisenberg groups" (other link).
Pictures and
animations (Up)
|
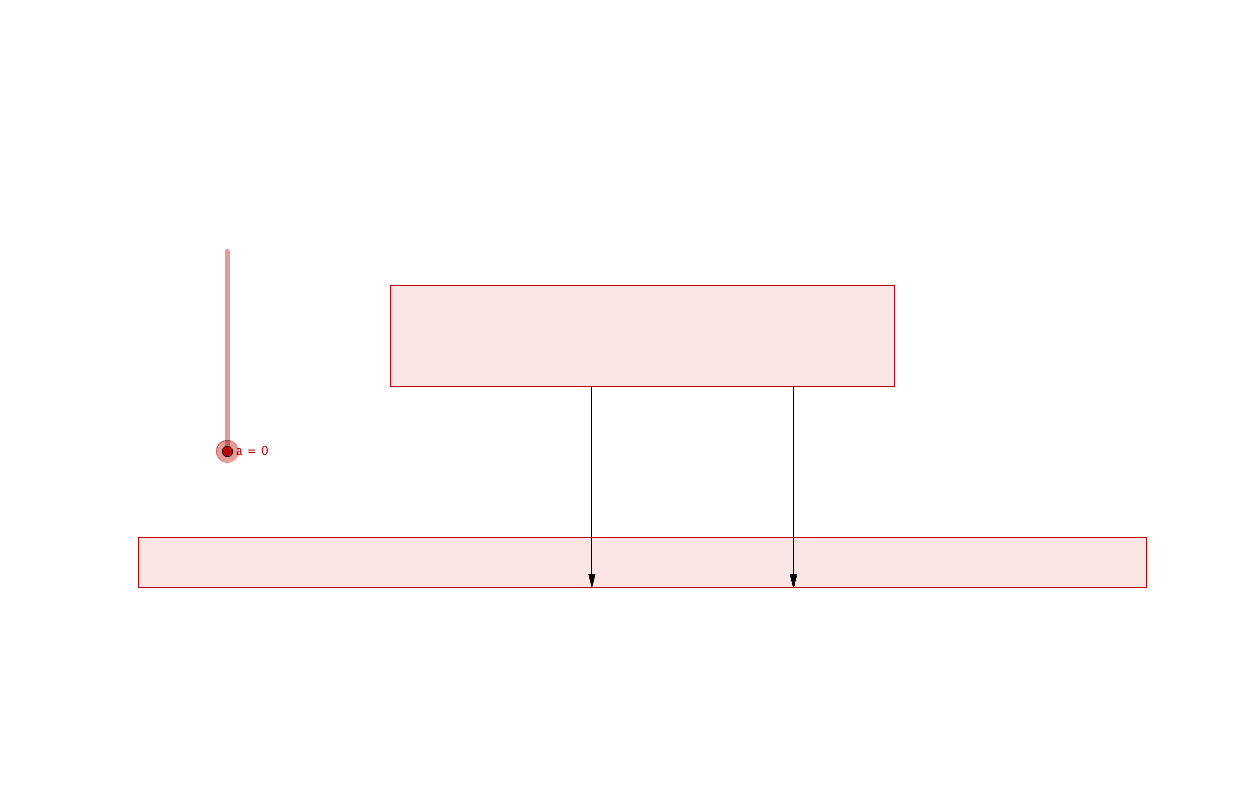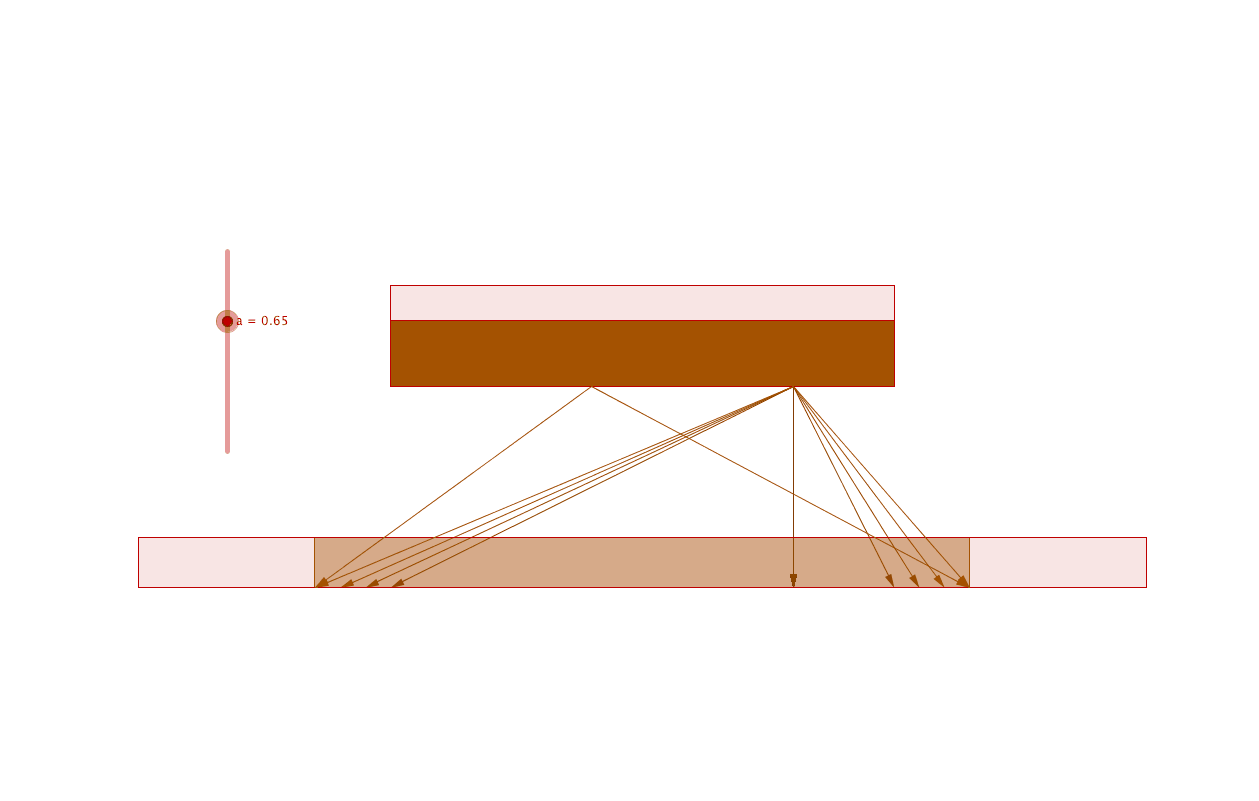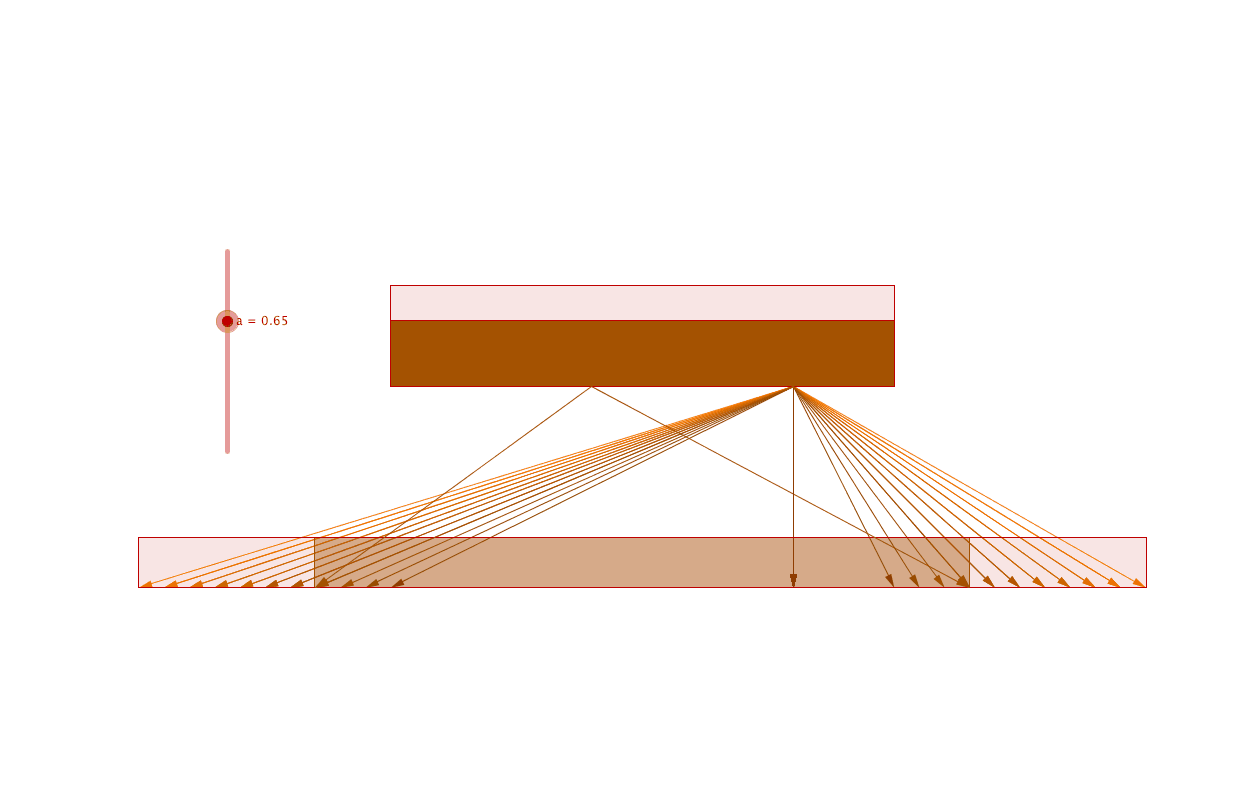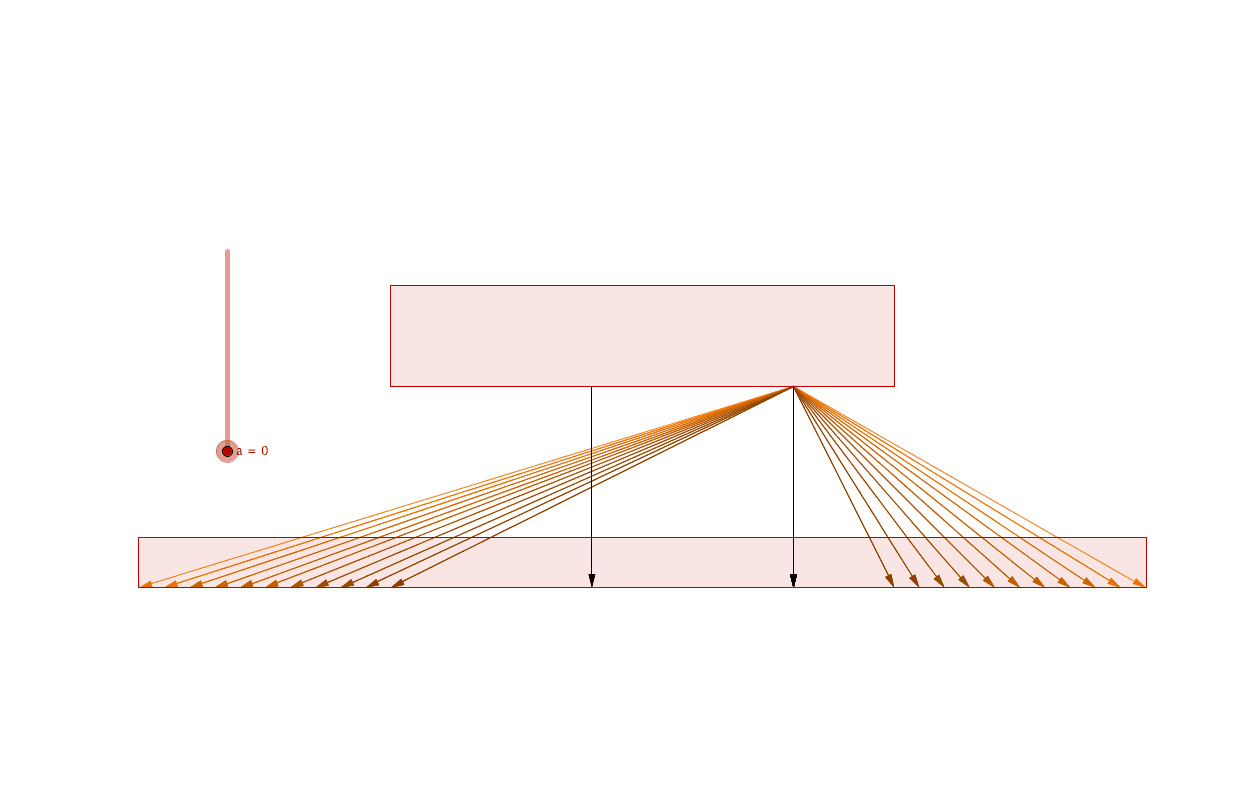
|
I have written some animations with Geogebra: In this animation,
you can examine the shape of the triangles of the Heisenberg group. The second animation
shows how the third coordinate of an horizontal
curve evolves with respect to the swept algebraic area.
Another one: animation.
On the left, the .gif file illustrate the "sunset coupling" of between two uniform measures defined in this paper .pdf. In this animation one can experiment several shadow couplings between a discrete measure (three atoms) and a continuous measure. Here are the sunset coupling, the left-curtain coupling and the middle-curtain coupling between two uniform measures.
|
Talks, surveys and slides (Up)
A list of talks with sometimes a survey in proceedings. I've added some slides: these are not "self-contained" diaporamas but rather the companions of a talk with chalk at blackboard.
- Vienna, "Optimal transport for Complex Data", July 2025
- Bordeaux, "Rencontre entre probabilistes", July 2024
- Munster (France 68), Kick-of of the ANR SOCOT, January 2024
- Champs-sur-Marne, "New Monge Problems and Applications", September 2024
- Dijon, séminaire SPOC, June 2023
- Oberwolfach, Heat Kernels, Stochastic Processes and Functional Inequalities, November 2022
- Bonn, New Trends in Dirichlet forms and Optimal Transport, September 2022
- Grenoble, AMS-EMS-SMF Meeting - special session on "Quantitative Geometry of Transportation Metrics", July 2022
- Paris, séminaire "Analyse-proba", April 2022
- Banff, Stochastic Mass Transport, March 2022
- Paris, "2e journée MAS MODE de la la SMAI", March 2022
- Münster, Oberseminar Stochastik, December 2021
- Marseille, "Schrödinger Problem and Mean-field PDE Systems", November 2021
- Kristiansand, Young researchers between geometry and stochastic analysis, June 2021
- Mulhouse, séminaire de mathématiques, December 2020
- Lorraine, séminaire généraliste de l'équipe probabilités et statistiques, December 2020
- Lille, séminaire de probabilités et statistique, November 2020
- Grenoble, séminaire de probabilités, March 2020
- Bergen, Young researchers between geometry and stochastic analysis, February 2020
- Osaka, Probability and Geometry, September 2019
- Bielerhöhe, summer school on stochastic and geometric analysis, July 2019
- London, stochastic analysis seminar, Jun 2019
- Vienna, probability seminar, February 2019
- Lyon, séminaire EDP-Analyse, November 2018
- Toulouse, séminaire "mathématiques de la décision", October 2018
- Toulouse, séminaire de probabilités, October 2018
- Marseille, séminaire de probabilités et statistique, September 2018
- Tours, "journées de probabilités", June 2018
- Bâle, Seminar in Analysis and Probability theory, May 2018
- Durham, Yorshire and Durham Geometry Day, March 2018
- Durham, Geometry and Topology seminar, March 2018
- Durham, Study group on optimal transport, March 2018
- Paris 5, Groupe de travail en probabilités du MAP5, October 2017 (.pdf)
- Grenoble, Journée sous-Riemanniennes 2017, October 2017 (video)
- Bordeaux, Journées du GDR AFHP, October 2017
- Bonn, IAP on Metric Measure Spaces and Ricci curvature, September 2017
- Oxford, Martingale Optimal Transport (and friends), September 2017
- Luxembourg, conference GeoProb2017, July 2017
- Nancy, séminaire de probabilités et statistiques, March 2017
- Jyväskylä (Finland), geometry seminar, February 2017
- Nancy, séminaire de géométrie différentielle, December 2016
- Bonn, follow-up workshop to JTP "optimal transportation", August 2016 (video)
- Orléans, séminaire du MAPMO, March 2016
- Paris, "groupe de travail courbure, transport optimal et probabilités", February 2016
- Montpellier, séminaire Darboux, January 2016
- Zürich, "Frontiers in analysis and probability", October 2015
- Nantes, séminaire de géométrie, September 2015
- Paris, séminaire d'analyse fonctionnelle, June 2015
- Grenoble, séminaire de probabilités, Mai 2015
- Bonn, workshop "Optimal transport and stochastics", March 2015 (video)
- Bonn, conference "New trends in optimal transport", March 2015
- Bordeaux, séminaire d'analyse, February 2015
- Marseille, groupe de travail Calcul des variations et EDP, February 2015
- Paris, thematic day "Optimal transport" of the subRiemannian trimestre, October 2014
- Palaiseau, groupe de travail de mathématiques financières de l'école polytechnique, October 2014 (.pdf)
- Luxembourg, probability seminar, October 2014
- Paris, conference on "Geometric analysis on subRiemannian manifolds", October 2014
- Bordeaux, conférence ProbaGéo, June 2014
- Karlsruhe, "5th Geometry day of Heidelberg-Karlsruhe-Strasbourg", June 2014
- Moscou, "International Youth Conference in Geometry and Control", April 2014 (video)
- Eindhoven, YEP XI "Young European Probabilists", March 2014
- Rennes, séminaire "probabilités et modèles aléatoires", March 2014
- Evry, séminaire "probabilités et maths financières", January 2014
- Bonn, Arbeitsgruppe Sturm, July 2013
- Orléans, "journées de probabilités", June 2013
- Marseille CIRM, rencontre "ANR Géométrie et transport", May 2013
- Toulon, séminaire "analyse non-linéaire et modélisation", December 2012
- Toulouse, séminaire de géométrie, December 2012
- Nice, séminaire "géométrie et analyse", December 2012
- Paris, séminaire "géométrie sous-riemannienne", December 2012
- Pisa, "ERC Workshop on Optimal transport theory" (short talk), November 2012 (.pdf)
- Dijon, journée "Contrôle optimal géométrique et transport optimal", septembre 2012
- Montpellier, rencontre "ANR Géométrie et transport", June 2012
- Poitiers, ''Conference ProbaGeo'', June 2012
- München, "Perspectives in Optimal transport", December 2011 (.pdf)
- Bonn, Intern. Conf. on Stoch. Anal. and App. (invited
session), September 2011
- Wien, Seminar Finanzmathematik, May 2011
- Dijon, séminaire "Statistiques, probabilités et applications",
May 2011
- Dijon, Conference in Memory of Paul Malliavin, October 2010
- Nice, "New trends in subRiemannian geometry", April 2010
- Maynooth (Ireland), colloquium, March 2010 (.pdf)
- IHES, "Analysis and geometry on groups", September 2009
- Grenoble, conférence interdisciplinaire "Repère et espace",
April 2009. Text for the proceedings, in french (.pdf).
- Marseille, séminaire d'analyse appliquée, March 2009
- Strasbourg, séminaire "GT3", January 2009. I've never used these slides! In french (.pdf)
- Nice, séminaire "Géométrie et analyse", January 2009
- Orsay, séminaire d'analyse harmonique, March 2009
- Kyoto, contributed talk in the conference "Probabilistic
approach to Geometry", August 2008. This survey is published in
the conference proceedings of the ASPM
57 . (.pdf)
- Luxembourg, mathematics seminar, June 2008
- CIRM (French mathematical center in Marseille), workshop
"Espaces métriques mesurés, courbure de Ricci minorée et
transport optimal", March 2008
- Nantes, séminaire de géométrie, January 2008
- Bologna, seminario di analisi matematica, November 2007
- Lausanne, séminaire de géométrie et théorie ergodique,
September 2007
- Levico Terme (close to Trento), conference "Analysis and
Geometry in metric measure spaces", June 2007
- Orsay (Paris sud), séminaire d'analyse harmonique, Mai 2007
- Toulouse, groupe de travail "optimal transport", January 2007
- Grenoble, séminaire de théorie spectrale et géométrie, October
2006. The related survey was published in the"Actes du Séminaire de Théorie Spectrale et
Géometrie"of the Institut Fourier. (.ps)
Last updated September 2021.